Pedal Triangles

Rozina Essani

For this assignment I will construct a pedal triangle to an arbitrary triangle ABC and investigate how pedal triangles behave when set equal to the triangle ABC’s centroid, incenter, orthocenter, circumcenter, side and vertices.

First we construct the pedal triangle RST for triangle ABC and call the pedal point P. The pedal point P is the point of intersection of the perpendiculars of all three sides of triangle ABC. The sides of ABC are extended. R, S, T are the points where the perpendiculars meet on the sides of ABC. Joining RST we get the pedal triangle of ABC.

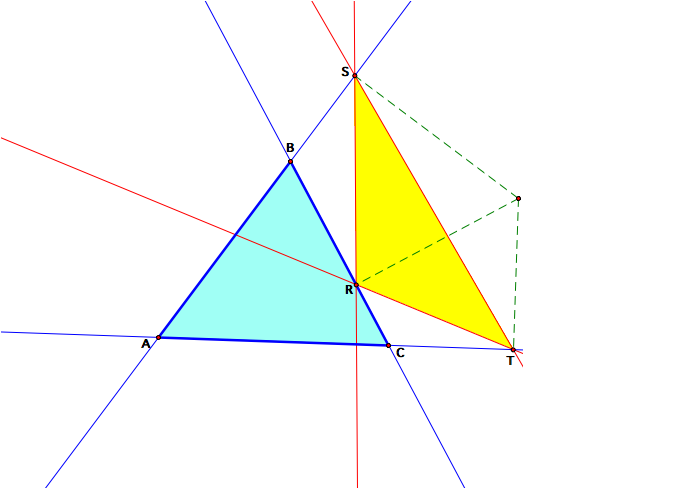
Triangle RST behaves in different ways as the pedal P is moved around. First we will see how RST behaves when it is set equal to the centroid of ABC.

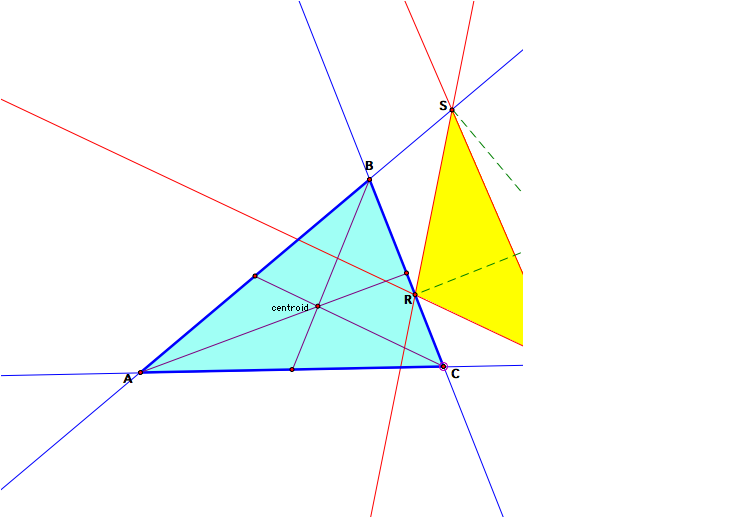
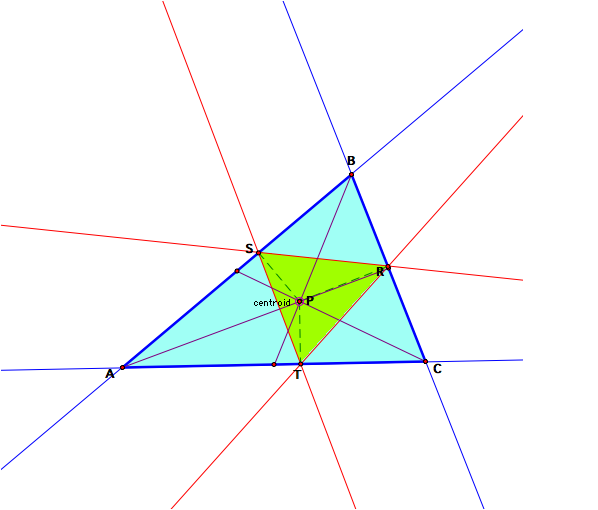
When P is set to equal the centroid of ABC, the pedal triangle RST is completely inside ABC. The only case where is is not is when ABC is an obtuse triangle.

Now lets look at when P is set equal to the incenter of ABC.

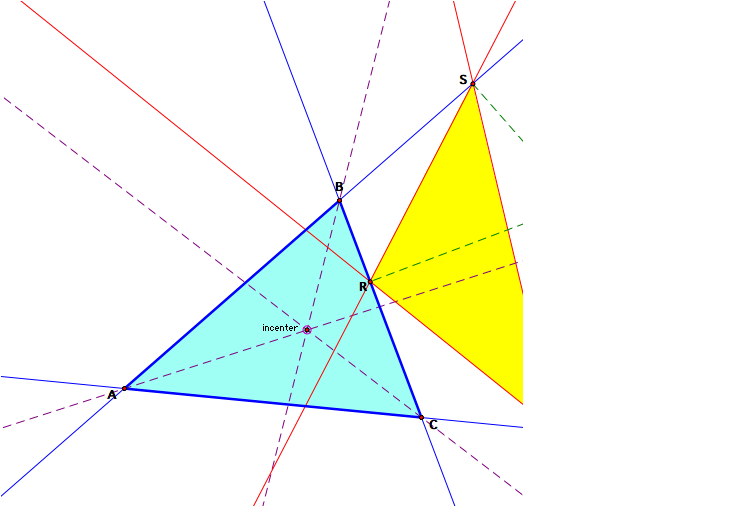
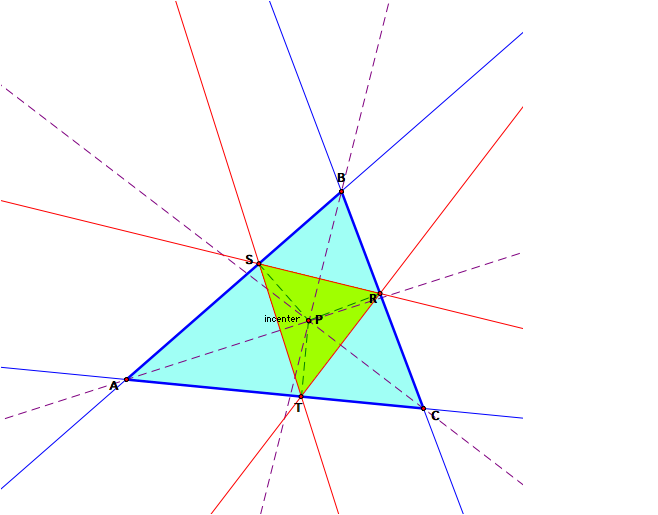
In the case where P is equal to the incenter, RST is completely inside ABC in all cases. In each case, obtuse, acute and right, the pedal triangle RST is close to an isosceles triangle.

Now we will investigate RST’s behavior when P is equal to the orthocenter of ABC.

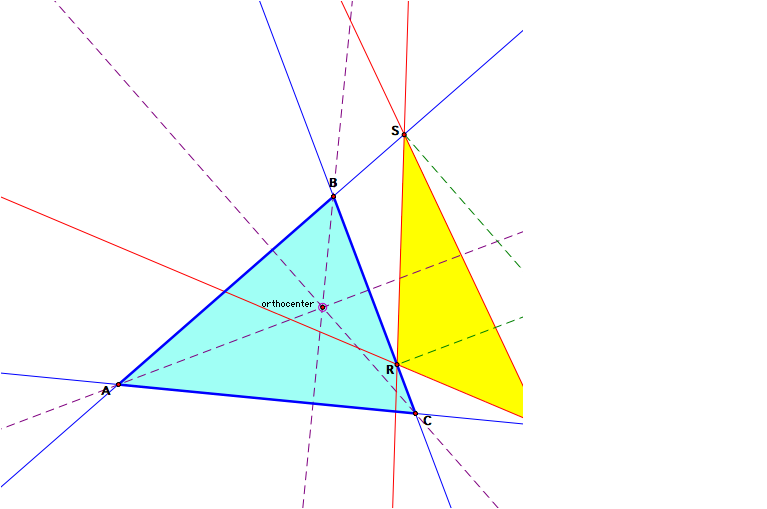
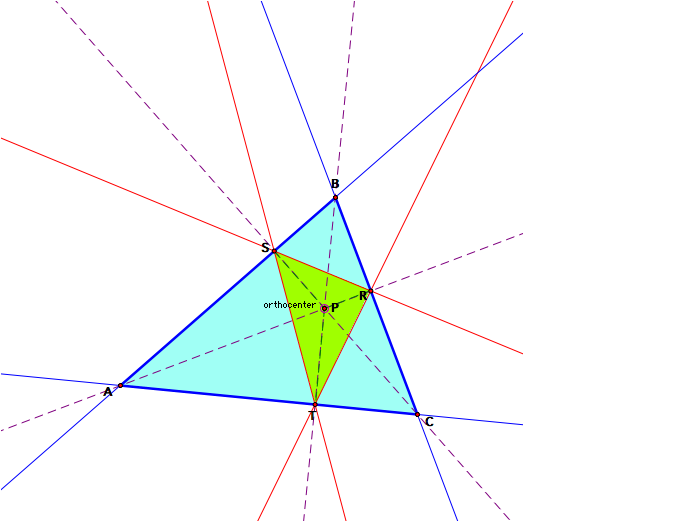
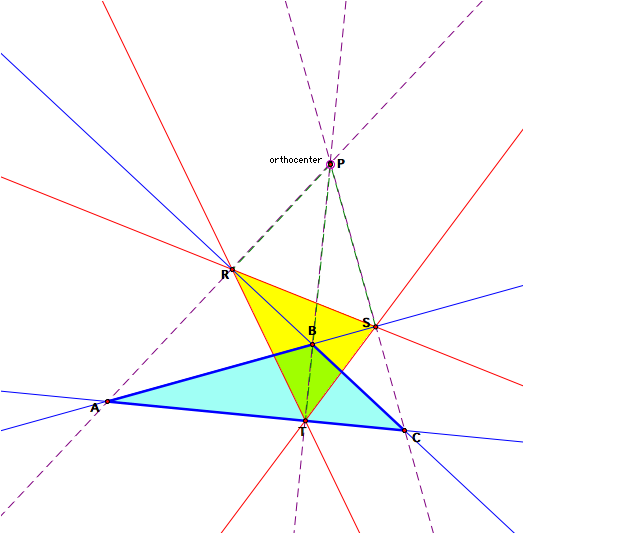
The orthocenter is constructed by finding the intersection of the altitudes of the vertices of ABC. When we set P equal to the orthocenter, RP, TP, and SP will always be parallel to AO, BO and CO respectively. When P is set equal to the orthocenter we can see that RP, TP and SP lies on AO, BO and CO.

Now we will work with the circumcenter of ABC.

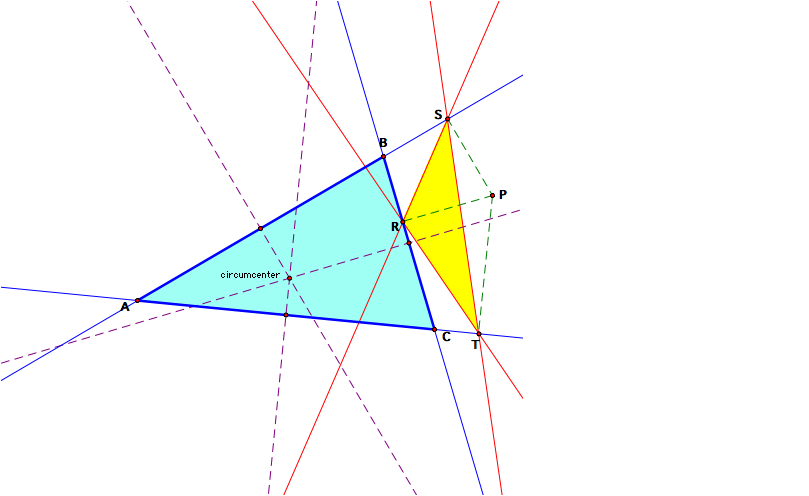
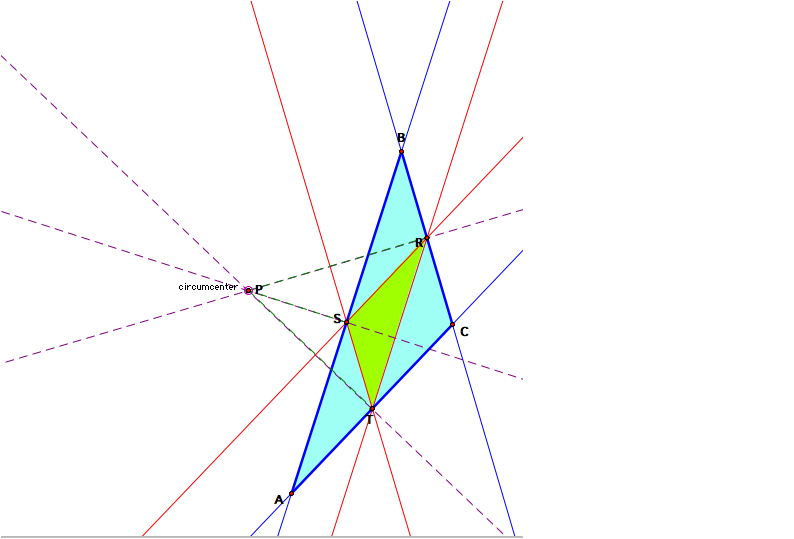
The circumcenter is the point of intersection of the perpendicular bisectors of the sides of ABC. When P is set equal to the circumcenter again RP, SP and TP lies on the perpendicular bisectors of ABC. But unlike the orthocenter scenario, RST is now completely inside ABC no matter if ABC is acute, obtuse or right. Also RST is congruent to ABC when P is equal to the circumcenter.

How does RST behave when P lies on a side and a vertex of ABC?

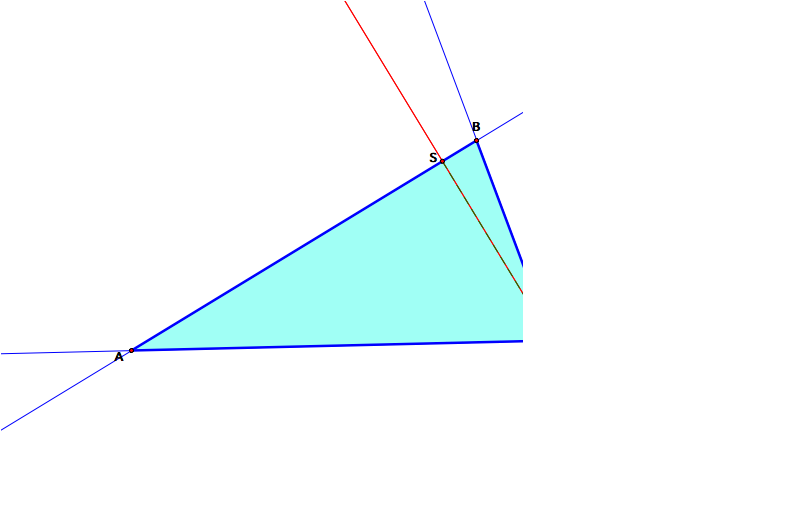
As we can see, when P lies on side, in this case on AC, P equals T and so the RST=RSP. If P moves to AB or BC then RST=PST and RST=RPS respectively.

In the case where P lies on a vertex (C) of ABC then P is equal to R and T, hence the triangle becomes a line with endpoints S and R=T=P=C. When P=B then we have a line with endpoints T and P=S=T=B. Same goes for when P=A.